Fourth body gravitation effect on the resonance orbit characteristics of the restricted three-body problem

Abstract

In this paper, the gravitational effect of a fourth body on the resonance orbit defined in the restricted three-body problem (RTBP) is considered. In this regard, Resonance Hamiltonian of the RTBP and the Hamiltonian associated with the fourth gravitational body that perturbs the resonance orbit are computed. The Melnikov approach is utilized as a mean for the detection of chaos in resonance orbit under the influence of the fourth gravitation body. In addition, the numerical simulation of RTBP and bicircular four-body model, time–frequency analysis (TFA), and fast Lyapunov indicator (FLI) are performed to verify the results of the Melnikov approach. The results indicate that for the (2:1) resonance orbit, the Melnikov integral computed over outer loop of separatrix does not cross the zero line, and consequently chaos is unexpected. On the other hand, the Melnikov integral computed over the inner sepratrix loop crosses the zero line indicating a potential for chaos. Similarly, it is shown that inclusion of the fourth body gravitation leads the (3:1) as well as the (4:1) resonance orbits to chaos. Additionally, simulation results indicate that for some initial conditions on the separatrix, the fourth body effect bounds the amplitude of the resonance orbits while diffusing its corresponding trajectory in the bounded phase space. TFA and the FLI verify similar results.

Appendix

Utilizing Newton’s gravitational law, one obtains that the motion of $P_1$ and $P_4$ in an inertial frame $(\xi _1, \xi _2)$ is described by the following equations:

$$\begin{aligned} \frac{\mathrm{d}^{2}\vec {\xi }_4}{\mathrm{d}t^{2}}&= Gm_1 \;\frac{\vec {\xi }_1 -\vec {\xi }_4}{\left| {\vec {\xi }_1 -\vec {\xi }_4}\right| ^{3}}+ Gm_2 \frac{\vec {\xi }_2 -\vec {\xi }_4}{\left| {\vec {\xi }_2 -\vec {\xi }_4}\right| ^{3}}\nonumber \\&+\,Gm_3\frac{\vec {\xi }_3 -\vec {\xi }_4}{\left| {\vec {\xi }_3 -\vec {\xi }_4}\right| ^{3}}\end{aligned}$$

(27)

$$\begin{aligned} \frac{\mathrm{d}^{2}\vec {\xi }_1}{\mathrm{d}t^{2}}&= Gm_2\;\frac{\vec {\xi }_2 -\vec {\xi }_1}{\left| {\vec {\xi }_2 -\vec {\xi }_1}\right| ^{3}}+ Gm_3 \frac{\vec {\xi }_3 -\vec {\xi }_1}{\left| {\vec {\xi }_3 -\vec {\xi }_1}\right| ^{3}}\nonumber \\&+\,Gm_4\frac{\vec {\xi }_4 -\vec {\xi }_1}{\left| {\vec {\xi }_4 -\vec {\xi }_1}\right| ^{3}} \end{aligned}$$

(28)

Defining the Earth centric frame ($P_1$), the following relative distance are defined:

$$\begin{aligned} \vec {r}_4 = \vec {\xi }_4 -\vec {\xi }_1, \quad \vec {r}_3 = \vec {\xi }_3 -\vec {\xi }_1, \quad \vec {r}_2 = \vec {\xi }_2 -\vec {\xi }_1 \end{aligned}$$

(29)

Thus, the equation of motion for $P_4$ will be

$$\begin{aligned} \frac{\mathrm{d}^{2}\vec {r}_4}{\mathrm{d}t^{2}}&= -\mu \frac{\vec {r}_4}{\rho _4^3}+ \varepsilon \left[ {-\frac{\vec {r}_2}{\rho _2^3}+\frac{\vec {r}_2 -\vec {r}_4}{\left| {\vec {r}_2 -\vec {r}_4}\right| ^{3}}}\right] \nonumber \\&+\, gm_3 \left[ {-\frac{\vec {r}_3}{\rho _3^3}+\frac{\vec {r}_3 -\vec {r}_4}{\left| {\vec {r}_3 -\vec {r}_4}\right| ^{3}}}\right] , \end{aligned}$$

(30)

where

$$\begin{aligned} \mu&= G(m_1 +m_4)\hbox { and}\\ \varepsilon&= Gm_2 \end{aligned}$$

Subsequently, (30) can be rewritten as

$$\begin{aligned} \frac{\mathrm{d}^{2}\vec {r}_4}{\mathrm{d}t^{2}}&= -\mu \frac{\vec {r}_4}{\rho _4^3}+ \varepsilon \left[ {-\frac{\vec {r}_2}{\rho _2^3}+\frac{\vec {r}_2 -\vec {r}_4}{\left| {\vec {r}_2 -\vec {r}_4}\right| ^{3}}}\right] \nonumber \\&+\gamma \left[ {-\vec {r}_3 +R_s^3 \frac{\vec {r}_3 -\vec {r}_4}{\left| {\vec {r}_3 -\vec {r}_4}\right| ^{3}}}\right] , \end{aligned}$$

(31)

where $\gamma = \frac{gm_3}{R_s^3}$

It can be shown that the perturbation terms in the RHS of (31) can be simplified as,

$$\begin{aligned}&-\frac{\vec {r}_2}{\rho _2^3}+\frac{\vec {r}_2 -\vec {r}_4}{\left| {\vec {r}_2 -\vec {r}_4}\right| ^{3}} = \frac{\partial }{\partial r_4}\left( {-\frac{\vec {r}_2\cdot \vec {r}_4}{\rho _2^3}+\frac{1}{\left| {\vec {r}_2 -\vec {r}_4}\right| }}\right) \nonumber \\&-\vec {r}_3 \!+\!R_s^3 \frac{\vec {r}_3 \!-\!\vec {r}_4}{\left| {\vec {r}_3 -\vec {r}_4}\right| ^{3}} \!=\! \frac{\partial }{\partial r_4 } \left( {-\vec {r}_3\cdot \vec {r}_4 +R_s^3 \frac{1}{\left| {\vec {r}_3 -\vec {r}_4}\right| }}\right) \nonumber \\ \end{aligned}$$

(32)

Finally, the equation of motion and the Hamiltonian of the negligible mass (P4) in the bicircular model will be

$$\begin{aligned}&\frac{\mathrm{d}^{2}\vec {r}_4}{\mathrm{d}t^{2}} = -\mu \frac{\vec {r}_4}{\rho _4^3} -\varepsilon \frac{\partial R_1}{\partial \vec {r}_4}-\gamma \frac{\partial R_2}{\partial \vec {r}_4}\end{aligned}$$

(33)

$$\begin{aligned}&H = -\frac{\mu ^{2}}{2L^{2}}+\varepsilon R_1 +\gamma R_2, \end{aligned}$$

(34)

where

$$\begin{aligned} R_1&= \frac{\vec {r}_2\cdot \vec {r}_4}{\rho _2^3}-\frac{1}{\left| {\vec {r}_2 -\vec {r}_4}\right| ^{3}}\nonumber \\ R_2&= \vec {r}_3\cdot \vec {r}_4 -R_s^3 \frac{1}{\left| {\vec {r}_3 -\vec {r}_4}\right| ^{3}} \end{aligned}$$

(35)

One can also express $R_2$ in terms of the resonance variables. Note that $R_2$ can also be defined as posed in Eq. (3),

$$\begin{aligned} R_2 = \sum _{j = 2}^\infty {P_j (\cos S_2) \left( {\frac{\rho _4}{\rho _3}}\right) ^{j}}, \end{aligned}$$

(36)

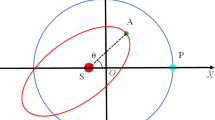
where $S_2$ is the angle between Earth–Satellite and Earth–Sun vectors, see Fig. 13. Similarly $\cos S_2$ can be defined:

$$\begin{aligned} \cos S_2&= \left( {1-\frac{1}{4}\gamma ^{2}}\right) \cos (\psi -\psi _S)\nonumber \\&+\frac{1}{4}\gamma ^{2}\cos (\psi +\psi _S -2\Omega ) \end{aligned}$$

(37)

Substitution of (37) into (36) with expansion of the trigonometric parts in terms of the Delaunay variables will result in the perturbation Hamiltonian $R_2$ in terms of resonance variables. In Eq. (38), the Hamiltonian associated with the fourth body mass is shown, assuming a zero inclination orbit:

$$\begin{aligned} \mathrm{Ham}_0 = \frac{1}{16384}\frac{1}{\rho _4^4}\left( {\begin{array}{l} -8960\Gamma R_S^3 \rho _4^4 \cos \left( {4\varpi _4 +4f_4 -4\varpi _3 -4f_3}\right) \\ -5120\Gamma R_S^3 \rho _4^4 \cos \left( {2\varpi _4 +2f_4 -2\varpi _3 -2f_3}\right) \\ -10240\Gamma R_S^3 \rho _4^3 \rho _3 \cos \left( {3\varpi _4 +3f_4 -3\varpi _3 -3f_3}\right) \\ -6144\Gamma R_S^3 \rho _4^3 \rho _3 \cos \left( {\varpi _4 +f_4 -\varpi _3 -f_3}\right) \\ -2304\Gamma R_S^3 \rho _4^4 -12288\Gamma R_S^3 \rho _4^2 \rho _3^2 \cos \left( {2\varpi _4 +2f_4 -2\varpi _3 -2f_3}\right) \\ -4096\Gamma R_S^3 \rho _4^2 \rho _3^2 \\ \end{array}}\right) \end{aligned}$$

(38)

Expressing orbital parameters using Delaunay variables and assuming zero eccentricity for the Sun’s orbit and equating higher orders of $\Phi$ and $\Psi$ to zero will yield (39).

Fig. 13

Geometry of the bicircular four-body problem ($P_1 =$ Earth, $P_2 =$ Moon, $P_3 =$ Sun, $P_4 =$ Satellite)

$$\begin{aligned}&\!\!\!\mathrm{Ham}: = -\frac{1}{16384}\frac{1}{\rho _4^4}\nonumber \\&\left( {\begin{array}{l} \left( {\begin{array}{l} 73728\rho _4^2 \cos \left( {2n_S t+2l_4 -2\phi -2t}\right) s^{2}\Psi ^{2}\Phi ^{2} \\ \quad +73728\sqrt{\frac{\Psi s^{\prime }\left( {-2s\Psi -s^{\prime }\Psi +2\Phi }\right) }{\left( {-s\Psi +\Phi }\right) ^{2}}}\,.\,\rho _4^2 \cos \left( {\frac{-2s^{\prime }t-\psi -s\phi +2n_s ts^{\prime }+2l_4 s^{\prime }-3\phi s^{\prime }}{s^{\prime }}}\right) s^{2}\Psi ^{2}\Phi ^{2} \\ \quad -14592\Phi ^{2}\Psi ^{2}s^{\prime 2}\sqrt{\frac{\Psi s^{\prime }\left( {-2s\Psi -\Psi s^{\prime }+2\Phi }\right) }{\left( {-s\Psi +\Phi }\right) ^{2}}}\rho _4^2 \cos \left( {\frac{2l_4 s^{\prime }-2s^{\prime }t+3\psi +3s\phi +s^{\prime }\phi +2n_s ts^{\prime }}{s^{\prime }}}\right) \\ \quad -36864\Psi ^{2}s^{\prime }\rho _4^2 s\Phi ^{2}-403200\Psi ^{2}s^{\prime 2}\rho _4^2 \cos \left( {2n_s t+2l_4 -2\phi -2t}\right) \Phi ^{2} \\ \quad -87552\Phi ^{2}\Psi ^{2}s^{\prime }\sqrt{\frac{\Psi s^{\prime }\left( {-2s\Psi -\Psi s^{\prime }+2\Phi }\right) }{\left( {-s\Psi +\Phi }\right) ^{2}}}\rho _4^2 \cos \left( {\frac{2l_4 s^{\prime }-2s^{\prime }t+3\psi +3s\phi +s^{\prime }\phi +2n_s ts^{\prime }}{s^{\prime }}}\right) s \\ \quad -147456\Psi ^{2}s^{\prime }\rho _4^2 \cos \left( {\frac{2\left( {n_S ts^{\prime }+l_4 s^{\prime }-s^{\prime }t+\psi +s\phi }\right) }{s^{\prime }}}\right) s\Phi ^{2} \\ \quad -32256\Phi ^{2}\Psi ^{2}s^{\prime }\sqrt{\frac{\Psi s^{\prime }\left( {-2s\Psi -\Psi s^{\prime }+2\Phi }\right) }{\left( {-s\Psi +\Phi }\right) ^{2}}}\rho _4^2 \cos \left( {\frac{1}{s^{\prime }}\left( {-2s^{\prime }t+5\psi +5s\phi +2n_S ts^{\prime }+2l_4 s^{\prime }+3\phi s^{\prime }}\right) }\right) s \\ \quad +294912\Phi ^{2}\Psi ^{2}s^{\prime }\sqrt{\frac{\Psi s^{\prime }\left( {-2s\Psi -\Psi s^{\prime }+2\Phi }\right) }{\left( {-s\Psi +\Phi }\right) ^{2}}}\rho _4^2 \cos \left( {\frac{1}{s^{\prime }}\left( {-2s^{\prime }t-3\psi -3s\phi -5\phi s^{\prime }+2n_S ts^{\prime }+2l_4 s^{\prime }}\right) }\right) s \\ \quad +205824\Psi ^{2}s^{\prime 2}\rho _4^2 \cos \left( {\frac{2\left( {n_S ts^{\prime }+s^{\prime }l_4 -s^{\prime }t+\psi +s\phi }\right) }{s^{\prime }}}\right) \Phi ^{2} \\ \quad +207360\Psi ^{2}s^{\prime }\rho _4^2 \cos \left( {2n_S t+2l_4 -2\phi -2t}\right) s\Phi ^{2} \\ \quad -77568\Phi ^{2}\Psi ^{2}s^{\prime 2}\sqrt{\frac{\Psi s^{\prime }\left( {-2s\Psi -\Psi s^{\prime }+2\Phi }\right) }{\left( {-s\Psi +\Phi }\right) ^{2}}}\rho _4^2 \cos \left( {\frac{-2s^{\prime }t-\psi -s\phi +2n_S ts^{\prime }+2l_4 s^{\prime }-3\phi s^{\prime }}{s^{\prime }}}\right) \\ \quad +49152\Phi ^{2}\Psi ^{2}s^{\prime 2}\sqrt{\frac{\Psi s^{\prime }\left( {-2s\Psi -\Psi s^{\prime }+2\Phi }\right) }{\left( {-s\Psi +\Phi }\right) ^{2}}}\rho _4^2 \cos \left( {\frac{-2s^{\prime }t-3\psi -3s\phi -5\phi s^{\prime }+2n_S ts^{\prime }+2l_4 s^{\prime }}{s^{\prime }}}\right) \\ \quad -221184\sqrt{\frac{\Psi s^{\prime }\left( {-2s\Psi -\Psi s^{\prime }+2\Phi }\right) }{\left( {-s\Psi +\Phi }\right) ^{2}}}\rho _4^2 \cos \left( {\frac{-2s^{\prime }l_4 -2s^{\prime }t+\psi +s\phi -\phi s^{\prime }+2n_S ts^{\prime }}{s^{\prime }}}\right) s^{2}\Psi ^{2}\Phi ^{2} \\ \quad +18432\Psi ^{2}s^{\prime }\rho _4^2 \cos \left( {\frac{2\left( {-s^{\prime }t-\psi -s\phi +n_S ts^{\prime }+s^{\prime }l_4 -2s^{\prime }\phi }\right) }{s^{\prime }}}\right) s\Phi ^{2} \\ \quad +346368\Psi ^{2}s^{\prime 2}\rho _4^2 \cos \left( {\frac{2\left( {-s^{\prime }t-\psi -s\phi +n_S ts^{\prime }+s^{\prime }l_4 -2s^{\prime }\phi }\right) }{s^{\prime }}}\right) \Phi ^{2} \\ \quad +69120\Phi ^{2}\Psi ^{2}s^{\prime }\sqrt{\frac{\Psi s^{\prime }\left( {-2s\Psi -\Psi s^{\prime }+2\Phi }\right) }{\left( {-s\Psi +\Phi }\right) ^{2}}}\rho _4^2 \cos \left( {\frac{2s^{\prime }l_4 -2s^{\prime }t+\psi +s\phi -\phi s^{\prime }+2n_S ts^{\prime }}{s^{\prime }}}\right) s \\ \quad -92160\Psi ^{2}s^{\prime 2}\rho _4^2 \cos \left( {\frac{2(s^{\prime }l_4 -s^{\prime }t-2\psi -2s\phi -3\phi s^{\prime }+n_S ts^{\prime })}{s^{\prime }}}\right) \Phi ^{2} \\ \quad +16128\Psi ^{2}s^{\prime 2}\rho _4^2 \cos \left( {\frac{2(n_S s^{\prime }t+l_4 s^{\prime }+3s\phi +2s^{\prime }\phi +3\psi -s^{\prime }t)}{s^{\prime }}}\right) \Phi ^{2} \\ \quad -49152\sqrt{\frac{\Psi s^{\prime }\left( {-2s\Psi -\Psi s^{\prime }+2\Phi }\right) }{\left( {-s\Psi +\Phi }\right) ^{2}}}\cos \left( {\frac{s\phi +s^{\prime }\phi +\psi }{s^{\prime }}}\right) \rho _4^2 s^{2}\Psi ^{2}\Phi ^{2}-6144\Psi ^{2}s^{\prime 2}\rho _4^2 \Phi ^{2} \\ \quad +36864\Psi ^{2}s^{\prime }\rho _4^2 \cos \left( {\frac{2\left( {s\phi +s^{\prime }\phi +\psi }\right) }{s^{\prime }}}\right) s\Phi ^{2}+6144\Psi ^{2}s^{\prime 2}\rho _4^2 \cos \left( {\frac{2\left( {s\phi +s^{\prime }\phi +\psi }\right) }{s^{\prime }}}\right) \Phi ^{2} \\ \quad +24576\rho _4^2 s^{2}\Psi ^{2}\Phi ^{2} \\ \quad +11520\Phi ^{2}\Psi ^{2}s^{\prime 2}\sqrt{\frac{\Psi s^{\prime }\left( {-2s\Psi -\Psi s^{\prime }+2\Phi }\right) }{\left( {-s\Psi +\Phi }\right) ^{2}}}\rho _4^2 \cos \left( {\frac{2l_4 s^{\prime }-2s^{\prime }t+\psi +s\phi -\phi s^{\prime }+2n_S ts^{\prime }}{s^{\prime }}}\right) \\ \quad -465408\Phi ^{2}\Psi ^{2}s^{\prime 2}\sqrt{\frac{\Psi s^{\prime }\left( {-2s\Psi -\Psi s^{\prime }+2\Phi }\right) }{\left( {-s\Psi +\Phi }\right) ^{2}}}\rho _4^2 \cos \left( {\frac{-2s^{\prime }t-\psi -s\phi +2n_S ts^{\prime }+2l_4 s^{\prime }-3\phi s^{\prime }}{s^{\prime }}}\right) s \\ \quad -5376\Phi ^{2}\Psi ^{2}s^{\prime 2}\sqrt{\frac{\Psi s^{\prime }\left( {-2s\Psi -\Psi s^{\prime }+2\Phi }\right) }{\left( {-s\Psi +\Phi }\right) ^{2}}}\rho _4^2 \cos \left( {\frac{-2s^{\prime }t+5\psi +5s\phi +2n_S ts^{\prime }+2l_4 s^{\prime }+3\phi s^{\prime }}{s^{\prime }}}\right) \\ \quad +32256\Psi ^{2}s^{\prime }\rho _4^2 \cos \left( {\frac{2\left( {-s^{\prime }t+2\psi +2s\phi +n_S ts^{\prime }+l_4 s^{\prime }+\phi s^{\prime }}\right) }{s^{\prime }}}\right) s\Phi ^{2} \\ \quad -54528\Psi ^{2}s^{\prime 2}\rho _4^2 \cos \left( {\frac{2\left( {-s^{\prime }t+2\psi +2s\phi +n_S ts^{\prime }+l_4 s^{\prime }+\phi s^{\prime }}\right) }{s^{\prime }}}\right) \Phi ^{2} \\ \quad \end{array}}\right) \cdot \Gamma R_S^3 \\ \\ \end{array}} \right) \end{aligned}$$

(39)